题目地址:https://leetcode.com/problems/out-of-boundary-paths/description/
题目描述
There is an m by n grid with a ball. Given the start coordinate (i,j) of the ball, you can move the ball to adjacent cell or cross the grid boundary in four directions (up, down, left, right). However, you can at most move N times. Find out the number of paths to move the ball out of grid boundary. The answer may be very large, return it after mod 10^9 + 7.
Example 1:
Input: m = 2, n = 2, N = 2, i = 0, j = 0
Output: 6
Explanation:
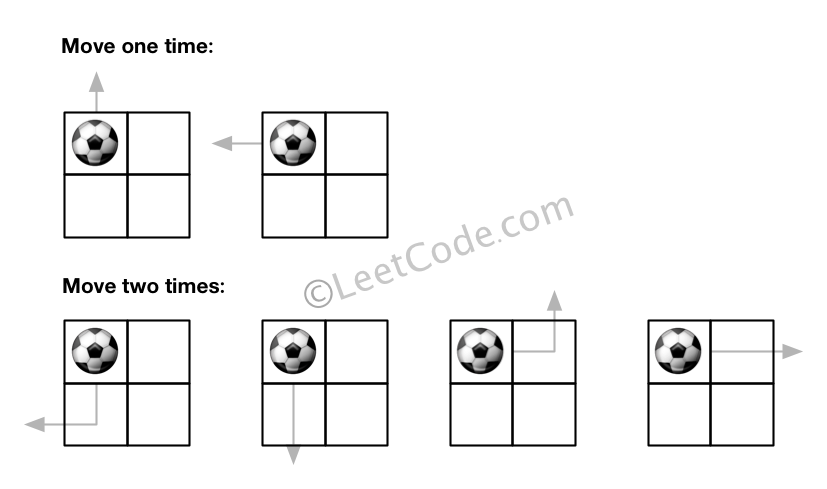
Example 2:
Input: m = 1, n = 3, N = 3, i = 0, j = 1
Output: 12
Explanation:
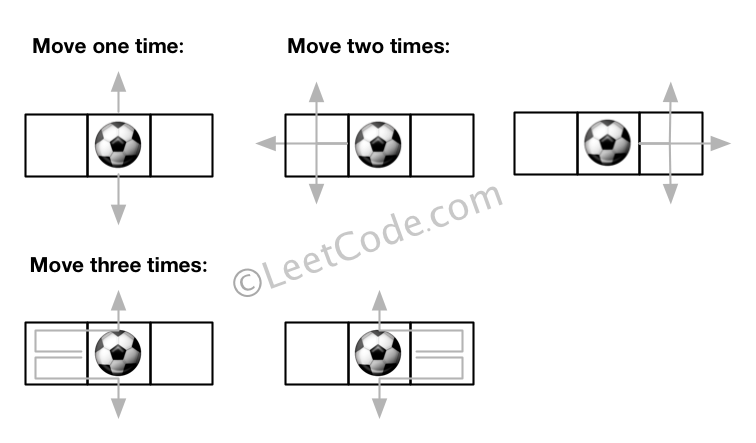
Note:
1、 Onceyoumovetheballoutofboundary,youcannotmoveitback.;
2、 Thelengthandheightofthegridisinrange[1,50].;
3、 Nisinrange[0,50].;
题目大意
每次可以把足球从一个格子移动到另一个格子,要求最多通过N步能使得球移动到外边的方案数?
解题方法
动态规划
这个题有个很明显的对于动态规划的提示,那就是要模10^9 + 7,也就是说结果会很大,普通的搜索可能hold不住。
使用三维数组dp[k][x][y]表示在不超过k步的情况下,从x,y点移动到外边需要的步数。那么,当前位置通过k步移动到外边的步数等于其周围4个位置走k - 1步移动到外边的步数和。
因为当x,y处于边界的时候,实际上只有两个或者三个相邻的位置,因为向边界方向走的话,只需要1步就可以移动到外部。所以,如果向当前位置的周围位置出界的话,那么从这个方向需要出去移动步数就是1.
最后求和取模。
时间复杂度是O(Nmn),空间复杂度是O(Nmn).
class Solution(object):
def findPaths(self, m, n, N, i, j):
"""
:type m: int
:type n: int
:type N: int
:type i: int
:type j: int
:rtype: int
"""
dp = [[[0] * n for _ in range(m)] for _ in range(N + 1)]
for s in range(1, N + 1):
for x in range(m):
for y in range(n):
v1 = 1 if x == 0 else dp[s - 1][x - 1][y]
v2 = 1 if x == m - 1 else dp[s - 1][x + 1][y]
v3 = 1 if y == 0 else dp[s - 1][x][y - 1]
v4 = 1 if y == n - 1 else dp[s - 1][x][y + 1]
dp[s][x][y] = (v1 + v2 + v3 + v4) % (10**9 + 7)
return dp[N][i][j]
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20
上面这个做法可以看出每个状态其实只和上一次的状态有关,因此可以做状态压缩节省空间。
只使用二维数组表示地图即可,需要注意的是每次循环的时候还是需要重新开一个全部为0的curStatus,为什么全部是0而不是dp的拷贝呢?因为我们每次对下一次的状态进行搜索之前,下个状态应该全部是未知的,我们下面的代码就是计算每个位置的值,因此不能初始化dp的拷贝,否则下面的代码不work。其实这个和上面的做法对比一下就知道了,因为上面的做法中,每一步开始的时候,里面的二维数组其实全部都是0.
每次搜索结束之后,需要更新dp,也就是我们把当前的状态作为下次搜索的初始状态。
时间复杂度是O(Nmn),空间复杂度是O(m*n).
class Solution(object):
def findPaths(self, m, n, N, i, j):
"""
:type m: int
:type n: int
:type N: int
:type i: int
:type j: int
:rtype: int
"""
dp = [[0] * n for _ in range(m)]
for s in range(1, N + 1):
curStatus = [[0] * n for _ in range(m)]
for x in range(m):
for y in range(n):
v1 = 1 if x == 0 else dp[x - 1][y]
v2 = 1 if x == m - 1 else dp[x + 1][y]
v3 = 1 if y == 0 else dp[x][y - 1]
v4 = 1 if y == n - 1 else dp[x][y + 1]
curStatus[x][y] = (v1 + v2 + v3 + v4) % (10**9 + 7)
dp = curStatus
return dp[i][j]
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22
状态搜索
这个dp其实属于对状态的搜索,如果看了《计算机考研机试指南》或者《挑战程序设计竞赛》的话,会很清楚的知道其实这是个搜索的题目。归根到底都是对状态的转移问题,所以这个方法的名称叫做动归还是搜索都可以。
这种的做法有点类似于BFS搜索的题目,我们在做BFS的时候也会记录当前处于哪一步,所以是非常类似的。我们定义了四个搜索的方向,从当前位置向周围4个方向进行搜索,如果搜索到了边界以外,和上面的做法类似的,我们把当前的步数+1;如果在边界以内,那么就把当前第s步的结果增加第s-1步的(nx, ny)位置能到达边界的解法步数。
时间复杂度是O(Nmn),空间复杂度是O(Nmn).
class Solution(object):
def findPaths(self, m, n, N, i, j):
"""
:type m: int
:type n: int
:type N: int
:type i: int
:type j: int
:rtype: int
"""
dp = [[[0] * n for _ in range(m)] for _ in range(N + 1)]
ds = [(0, 1), (0, -1), (-1, 0), (1, 0)]
for s in range(1, N + 1):
for x in range(m):
for y in range(n):
for d in ds:
nx, ny = x + d[0], y + d[1]
if nx < 0 or nx >= m or ny < 0 or ny >= n:
dp[s][x][y] += 1
else:
dp[s][x][y] = (dp[s][x][y] + dp[s - 1][nx][ny]) % (10**9 + 7)
return dp[N][i][j]
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22
同样的可以优化空间。
时间复杂度是O(Nmn),空间复杂度是O(m*n).
class Solution(object):
def findPaths(self, m, n, N, i, j):
"""
:type m: int
:type n: int
:type N: int
:type i: int
:type j: int
:rtype: int
"""
dp = [[0] * n for _ in range(m)]
ds = [(0, 1), (0, -1), (-1, 0), (1, 0)]
for s in range(1, N + 1):
curStatus = [[0] * n for _ in range(m)]
for x in range(m):
for y in range(n):
for d in ds:
nx, ny = x + d[0], y + d[1]
if nx < 0 or nx >= m or ny < 0 or ny >= n:
curStatus[x][y] += 1
else:
curStatus[x][y] = (curStatus[x][y] + dp[nx][ny]) % (10**9 + 7)
dp = curStatus
return dp[i][j]
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24
记忆化搜索
其实,应该是先有了记忆化搜索的代码才能推出dp。这个题我用记忆化搜索重新实现了一下,但是发现果然过不了啊!但是记忆化搜索确实能加深我们对这个题目的理解。
把上面的状态搜索的dp改成记忆化搜索后的代码如下。如何加深理解呢?看看dfs的参数,变量其实只有x,y两个。dfs函数代表了我们从(x, y)位置出发,最多移动N次的情况下能到达边界的个数。所以,我们的(x, y)的初始化值是题目要求的(i, j).
最后TLE了,很无奈,因为C++版本的能够通过。
时间复杂度是O(Nmn),空间复杂度是O(Nmn).
class Solution(object):
def findPaths(self, m, n, N, i, j):
"""
:type m: int
:type n: int
:type N: int
:type i: int
:type j: int
:rtype: int
"""
dp = [[[0] * n for _ in range(m)] for _ in range(N + 1)]
return self.dfs(m, n, N, i, j, dp)
def dfs(self, m, n, N, x, y, dp):
if N == 0:
return 0
if x < 0 or x >= m or y < 0 or y >= n:
return 1
if dp[N][x][y]:
return dp[N][x][y]
ds = [(0, 1), (0, -1), (-1, 0), (1, 0)]
for d in ds:
nx, ny = x + d[0], y + d[1]
dp[N][x][y] = (dp[N][x][y] + self.dfs(m, n, N - 1, nx, ny, dp)) % (10**9 + 7)
return dp[N][x][y]
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25
相似题目
688. Knight Probability in Chessboardopen in new window62. Unique Pathsopen in new window63. Unique Paths IIopen in new window913. Cat and Mouseopen in new window
参考资料
http://www.cnblogs.com/grandyang/p/6927921.html https://zxi.mytechroad.com/blog/dynamic-programming/leetcode-576-out-of-boundary-paths/
DDKK.COM 弟弟快看-教程,程序员编程资料站,版权归原作者所有
本文经作者:负雪明烛 授权发布,任何组织或个人未经作者授权不得转发