题目地址:https://leetcode.com/problems/count-primes/open in new window
Total Accepted: 36655 Total Submissions: 172606 Difficulty: Easy
题目描述
Count the number of prime numbers less than a non-negative number, n.
Example:
Input: 10
Output: 4
Explanation: There are 4 prime numbers less than 10, they are 2, 3, 5, 7.
题目大意
计算小于n的素数有多少个。
解题方法
素数筛法
http://blog.csdn.net/blitzskies/article/details/45442923open in new window 提示用Sieve of Eratosthenesopen in new window的方法。
素数筛法就是把这个数的所有倍数都删除掉,因为这些数一定不是素数。最后统计一下数字剩余的没有被删除的个数就好。
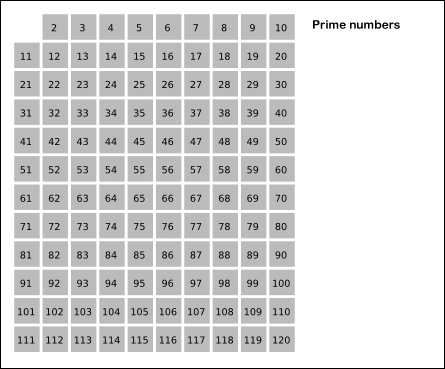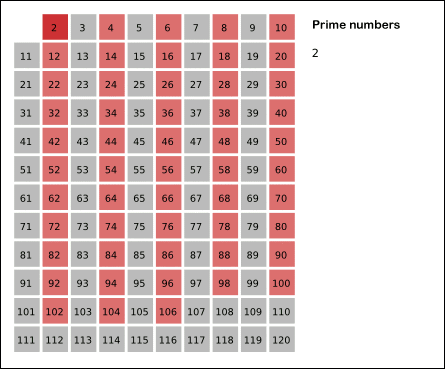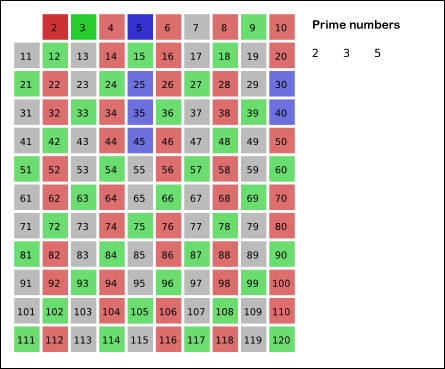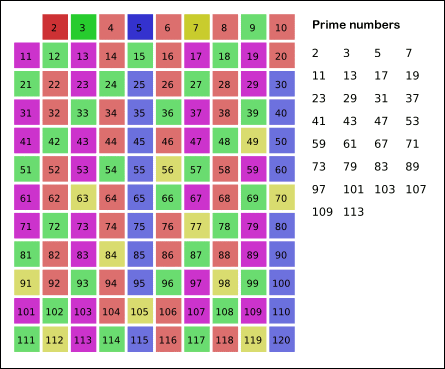
也是学习了。
Java解法。
重点是优化效率,每一步的效率都要优化。
用List都会效率低,最后用数组好了。
/**
* 统计质数的数目。使用数组,用列表效率低。
*
* @param n
* @return
*/
public static int countPrimes3(int n) {
//n个元素的数组,其实只用到了n-2个。多见了2个防止输入0的时候崩溃。
int[] nums = new int[n];
//把所有的小于n的大于2的数字加入数组里
for (int i = 2; i < n; i++) {
//注意计算质数从2开始的,而数组从0开始
nums[i - 2] = i;
}
//统一的长度,优化计算
int size = nums.length;
//各种优化效率,只计算到sqrt(n)
for (int i = 0; i * i < size; i++) {
//获取数组中的数字
int temp = nums[i];
//如果不是0,则进行计算,否则直接跳过,因为这个数已经是之前的某数字的倍数
if (temp != 0) {
//把该数字的倍数都删去,因为他们都不是质数
//int i1 = temp - 1 优化效率,是因为比如用5来删除数字的时候15=3*5已经被删除过了,所以从20=5*4开始删除
for (int i1 = temp - 1; i + temp * i1 < size; i1++) {
//如果这个数不是素数则被置0,置成其他的负数也一样,只是为了区分和统计
//i + temp * i1,i是因为从当前数字开始,比如10是从5的位置开始计算位置
nums[i + temp * i1] = 0;
}
}
}
return countNums(nums);
}
/**
* 计算数组中的0出现了多少次
*
* @param nums
* @return
*/
public static int countNums(int[] nums) {
int size = nums.length;
int zeros = 0;
for (int num : nums) {
if (num == 0) {
zeros++;
}
}
return size - zeros;
}
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45 46 47 48 49 50 51 52
没必要把所有的数字都保存到一个数组里面,可以直接记录和数字对应的位置的数字是不是质数。如果不是质数,则在对应位置保存true.最后统计不是true的,即质数的个数即可。
这个方法可以通用下去。类似的统计的题目只记录对应的位置是否满足条件,最后统计符合条件的个数。
/**
* 统计质数的数目。使用数组,用列表效率低。
*
* @param n
* @return
*/
public static int countPrimes4(int n) {
//n个元素的数组,其实只用到了n-2个。多见了2个防止输入0的时候崩溃。
boolean[] nums = new boolean[n];
//各种优化效率,只计算到sqrt(n)
for (int i = 2; i * i < n; i++) {
//获取数组中的数字是不是为0
//不是质数则为true
boolean temp = nums[i];
//如果不是true,说明不是质数,则进行计算,否则直接跳过,因为这个数已经是之前的某数字的倍数
if (!temp) {
//把该数字的倍数都删去,因为他们都不是质数
//int i1 = temp - 1 优化效率,是因为比如用5来删除数字的时候15=3*5已经被删除过了,所以从20=5*4开始删除
for (int j = i; i * j < n; j++) {
//如果这个数不是素数则被置true
//i + temp * i1,i是因为从当前数字开始,比如10是从5的位置开始计算位置
nums[i * j] = true;
}
}
}
return countNums2(nums);
}
/**
* 计算数组中的不是素数的false出现了出现了多少次
*
* @param nums
* @return
*/
public static int countNums2(boolean[] nums) {
int notZeros = 0;
for (int i = 2; i < nums.length; i++) {
if (!nums[i]) {
notZeros++;
}
}
return notZeros;
}
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22 23 24 25 26 27 28 29 30 31 32 33 34 35 36 37 38 39 40 41 42 43 44 45
二刷,使用Python解法,速度很慢,勉强通过了。
class Solution(object):
def countPrimes(self, n):
"""
:type n: int
:rtype: int
"""
nums = [True] * n
for i in xrange(2, n):
j = 2
while i * j < n:
nums[i * j] = False
j += 1
res = 0
for i in xrange(2, n):
if nums[i]:
res += 1
return res
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17
C++版本如下:
class Solution {
public:
int countPrimes(int n) {
vector<bool> nums(n, true);
for (int i = 2; i < n; i++) {
int j = 2;
while (i * j < n) {
nums[i * j] = false;
j ++;
}
}
int res = 0;
for (int i = 2; i < n; i++) {
if (nums[i]){
res ++;
}
}
return res;
}
};
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20
C++数组初始化需要使用memset,而且数组的大小n不能是0,数组解法如下。
class Solution {
public:
int countPrimes(int n) {
if (n <= 0) return false;
bool nums[n];
memset(nums, true, sizeof(nums));
for (int i = 2; i < n; i++) {
int j = 2;
while (i * j < n) {
nums[i * j] = false;
j ++;
}
}
int res = 0;
for (int i = 2; i < n; i++) {
if (nums[i]){
res ++;
}
}
return res;
}
};
1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16 17 18 19 20 21 22
DDKK.COM 弟弟快看-教程,程序员编程资料站,版权归原作者所有
本文经作者:负雪明烛 授权发布,任何组织或个人未经作者授权不得转发